Problem Statement

Given an array of integers nums and an integer k, return the total number of continuous subarrays whose sum equals to k.

Example 1

Input: nums = [1,1,1], k = 2
Output: 2

Example 2

Input: nums = [1,2,3], k = 3
Output: 2

Constraints

• 1 <= nums.length <= 2 * 10^4.

• -1000 <= nums[i] <= 1000.

• -10^7 <= k <= 10^7.

Solution 1: Bruteforce

For each element, for all subarrays starting from it, choose the satisfied ones.

Example 3

For nums = [1, -1, 0] and k = 0, you get 3 subarrays for the result:

• Three subarrays start from 1, which are [1], [1, -1], and [1, -1, 0]. Only the last two are satisfied.

• Two subarrays start from -1, which are [-1] and [-1, 0]. None is satisfied.

• Only [0] is the subarray starting from 0. It is satisfied.

Code

#include <iostream>
#include <vector>
using namespace std;
int subarraySum(vector<int>& nums, int k) {
    int count = 0;
    for (int i = 0; i < nums.size(); i++) {
        int sum = 0;
        for (int j = i; j < nums.size(); j++) {
            sum += nums[j];
            if (sum == k) {
                count++;
            }
        }
    }
    return count;
}
int main() {
    vector<int> nums{1,1,1};
    cout << subarraySum(nums, 2) << endl;
    nums = {1,2,3};
    cout << subarraySum(nums, 3) << endl;
    nums = {1,-1,0};
    cout << subarraySum(nums, 0) << endl;
}

Output:
2
2
3

Complexity

• Runtime: O(N^2), where N = nums.length.

• Extra space: O(1).

Solution 2: Prefix sum

In the solution above, many sums can be deducted from the previous ones.

Example 4

For nums = [1, 2, 3, 4]. Assume the sum of the subarrays [1], [1, 2], [1, 2, 3], [1, 2, 3, 4] were computed in the first loop. Then the sum of any other subarray can be deducted from those values.

• sum([2, 3]) = sum([1, 2, 3]) - sum([1]).

• sum([2, 3, 4]) = sum([1, 2, 3, 4]) - sum([1]).

• sum([3, 4]) = sum(1, 2, 3, 4) - sum(1, 2).

In general, assume you have computed the sum sum[i] for the subarray [nums[0], nums[1], ..., nums[i]] for all 0 <= i < nums.length. Then the sum of the subarray [nums[j], nums[j+1], ..., nums[i]] for any 0 <=j <= i can be computed as sum[i] - sum[j].

Code

#include <iostream>
#include <vector>
using namespace std;
int subarraySum(vector<int>& nums, int k) {
    vector<int> sum(nums.size());
    sum[0] = nums[0];
    for (int i = 1; i < nums.size(); i++) {
        sum[i] = sum[i-1] + nums[i];
    }
    int count = 0;
    for (int i = 0; i < nums.size(); i++) {
        if (sum[i] == k) {
            count++;
        }
        for (int j = 0; j < i; j++) {
            if (sum[i] - sum[j] == k) {
                count++;
            }
        }
    }
    return count;
}
int main() {
    vector<int> nums{1,1,1};
    cout << subarraySum(nums, 2) << endl;
    nums = {1,2,3};
    cout << subarraySum(nums, 3) << endl;
    nums = {1,-1,0};
    cout << subarraySum(nums, 0) << endl;
}

Output:
2
2
3

Complexity

• Runtime: O(N^2), where N = nums.length.

• Extra space: O(N).

Solution 3: Faster lookup

You can rewrite the condition sum[i] - sum[j] == k in the inner loop of Solution 2 to sum[i] - k == sum[j].

Then that loop can rephrase to "checking if sum[i] - k was already a value of some computed sum[j]".

Now you can use an unordered_map to store the sums as indices for the fast lookup.

Code

#include <iostream>
#include <vector>
#include <unordered_map>
using namespace std;
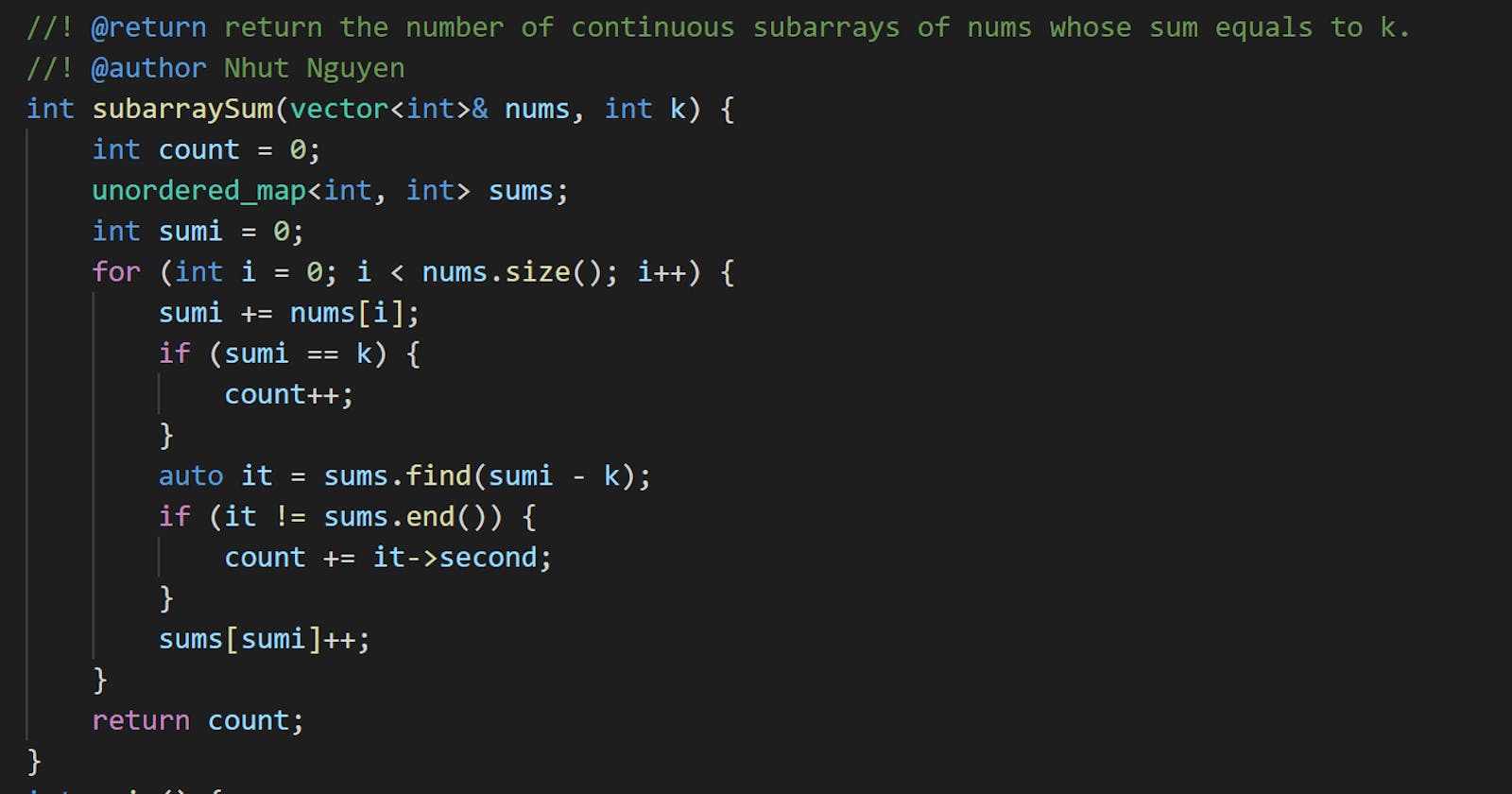
int subarraySum(vector<int>& nums, int k) {
    int count = 0;
    unordered_map<int, int> sums;
    int sumi = 0;
    for (int i = 0; i < nums.size(); i++) {
        sumi += nums[i];
        if (sumi == k) {
            count++;
        }
        auto it = sums.find(sumi - k);
        if (it != sums.end()) {
            count += it->second;
        }
        sums[sumi]++;
    }
    return count;
}
int main() {
    vector<int> nums{1,1,1};
    cout << subarraySum(nums, 2) << endl;
    nums = {1,2,3};
    cout << subarraySum(nums, 3) << endl;
    nums = {1,-1,0};
    cout << subarraySum(nums, 0) << endl;
}

Output:
2
2
3

Complexity

• Runtime: O(N), where N = nums.length.

• Extra space: O(N).

References

• https://leetcode.com/problems/subarray-sum-equals-k/

Thanks for reading. Feel free to share your thought about my content and check out my FREE book “10 Classic Coding Challenges”.